Symmetrical scale generators

One of the unique features of the Harmonia app are its score generators, which offer instructors a way to quickly generate and add a variety of musical scores to Harmonia documents for illustrating concepts during a lecture or for creating computer-gradable exercises for homework assignments. These in-app generators cover musical fundamentals such as pitches, intervals, scales, chords, and roman numerals, and more complex topics such as species counterpoint (new to fall 2021), as well as a wide variety of diatonic and chromatic SATB chord progressions.

New to fall 2021, Harmonia’s scale generators have been augmented to offer not only options for generating major, minor, and modal scales, but now also symmetrical collections such as whole tone, octatonic, and hexatonic scales.

Diatonic Scale Generators

Use our diatonic scale generators to quickly generate large numbers of major and minor scales and diatonic modes. Determine clefs and keys signatures (or allowable accidentals when key signatures are hidden), scale direction, and whether the octave note should be included (for collection vs scale exercises).

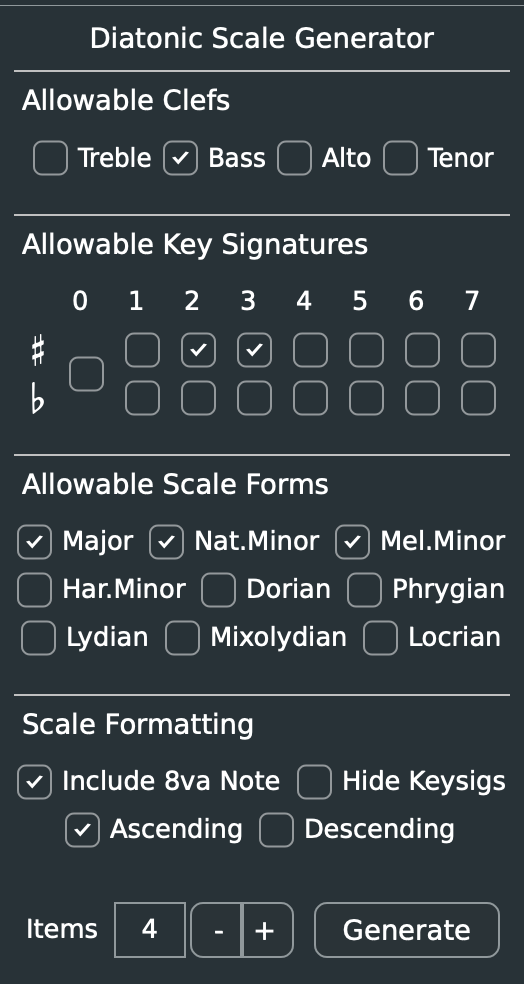
Generate one scale at a time or multiple scales within a single score, as seen below.

The single ascending B-flat Dorian scale the left is notated in treble clef with a key signature of 4 flats. The time signature is hidden. The following descending G Lydian scale on the right is notated in bass clef using accidentals instead of a key signature (which is hidden). The time signature is visible.

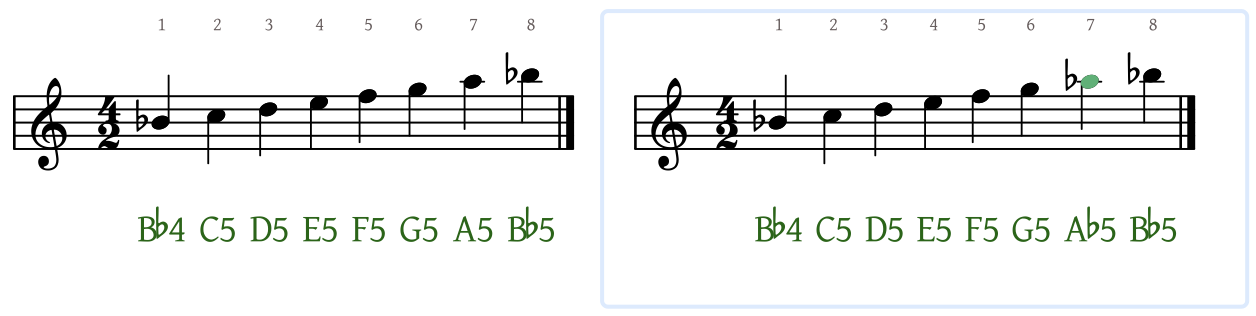
This alto-clef score contains four scales, all with different key signatures: an ascending G natural minor scale, a descending E-flat major scales, an ascending F Lydian scale, and an ascending C-sharp melodic minor scale.

Once generated and added to a Harmonia document, instructors can edit the notes on the staff to create even more scales if desired. Lower the A-natural of the auto-generated Lydian scale of the left to create a Lydian-Mixolydian scale on the right.

Symmetrical Scale Generators

New to our fall 2021 release is the ability to generate symmetrical scales, or scales that evenly divide the octave by a specific, symmetrical pattern. Instructors can use the generator settings to create any number of whole-tone, octatonic, or hexatonic scales in a variety of clefs.

Harmonia’s symmetric scale generators are labeled by scale type and the specific pitch-class(es) contained within, represented by integer notation. Integer notation is a method of representing pitch classes (pcs) as numbers. By tradition, C and all of its octave and enharmonic equivalents are represented by 0. C-sharp or D-flat is 1, D is 2, and so forth. We use the integers 0-11 to represent all the notes of the chromatic scale.

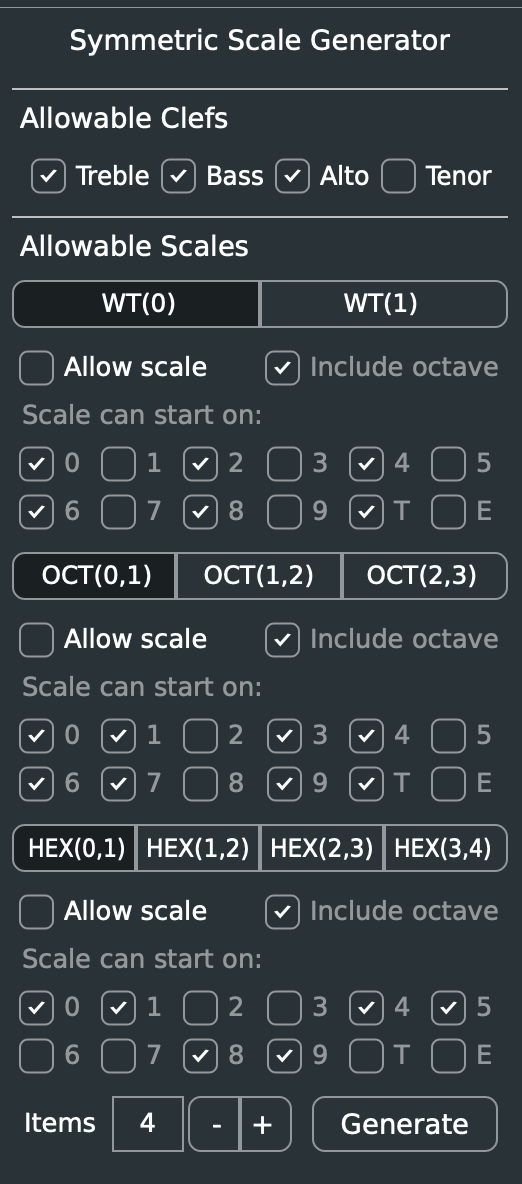
Whole-Tone Scales

Whole-tone scales are scales composed of only whole steps and are labeled WT(0) and WT(1) because each of these scales contains either pc0 (C) or pc1 (C-sharp or D-flat). These scales may start on any note, but all WT(0) scales contain only even-numbered pcs, <0-2-4-6-8-10-0> all WT(1) scales contain only odd-numbered pcs, <1-3-5-7-9-11-1>.

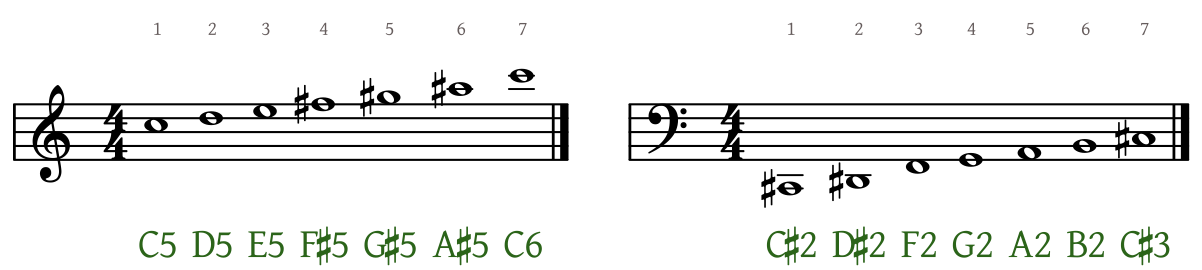
Octatonic Scales

Octatonic scales divide the octave symmetrically by alternating half steps (H) and whole steps (W), thus creating an eight-note scale with a H-W-H-W-H-W-H-W pattern. There are three unique octatonic scale collections named according to the smallest integers that make up a half-step interval. OCT(0,1) is so named because it contains pcs 0 and 1 (C and C#/Db) and is spelled <0-1-3-4-6-7-9-10-0>. OCT (1,2) contains pcs 1 and 2 (C#/Db and D) and is composed of <1-2-4-5-7-8-10-11-1> and OCT(2,3) contains pcs 2 and 3 (D and D#/Eb) and is spelled <2-3-5-6-8-9-11-0-2>. Each of these scales may begin on any pitch of their respective collection but must contain both identifying pc integers.

Hexatonic Scales

Hexatonic scales divide the octave symmetrically by alternating a half-step interval (H) with an interval of three half steps or a minor third (m3), thus creating a six-note scale with a H-m3-H-m3-H-m3 pattern. There are four unique hexatonic scale collections named according to the smallest integers that make up a half-step interval. HEX(0,1) contains pcs 0 and 1 (C and C#/Db) and is composed of <0-1-4-5-8-9-0>. HEX (1,2) contains pcs 1 and 2 (C#/Db and D) and is spelled <1-2-5-6-9-10-1>. HEX (2,3) contains pcs 2 and 3 (D and D#/Eb) and is spelled <2-3-6-7-10-11-2>. HEX (3,4) contains pcs 3 and 4 (D#/Eb and E) and is composed of <3-4-7-8-11-0-3>. Again, each of these scales may begin on any pitch of their respective collection but must contain both identifying pc integers.